A Triangle With a 45 Degrees Angle in Square : A very hard problem from Geometry

Let P and Q be the points where AN and AM intersect the diagonal BD, respectively. It is noted that ∠QAN=∠QDN=45∘.Thus, AQND is a cyclic quadrilateral, therefore implying that ∠AQN=90∘.

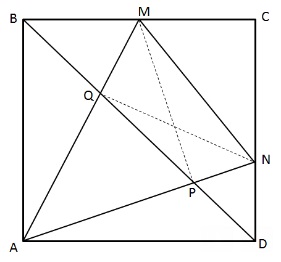
This in turn implies that ΔAQN is a right-isoceles triangle with AN as the hypotenuse. Thus, AQ=AN2–√.

The same argument can be employed to show that AP=AM2–√.

Now, since triangles APQ and AMN share a common vertex angle A, we have, Area(ΔAPQ)Area(ΔAMN)=AQ⋅APAM⋅AN. However, from the conclusions in the previous two paragraphs, we have AQAN=APAM=12–√.

This therefore implies that Area(ΔAPQ)Area(ΔAMN)=12, or equivalently, Area(ΔAPQ)Area(MNPQ)=1, as claimed by the problem.

The problem, due to V. Proizvolov, appeared in Kvant - a popular Russian magazine - (#1, 2004, M1895), with a solution in a later issue (#4, 2004).

Class IX West Bengal Board Ganit Prakash Chapter 20 Solutions- Co-ordinate Geometry

Class IX West Bengal Board Ganit Prakash Chapter 20 Solutions - Coordinate Geometry

In this pdf you will get all the solved problem from the exercise 20. Before going through the solutions you are advised to first try the problem yourself. If you get stuck, seek help from the solutions. Don't copy blindly. If you have any query, comment below.

Can you solve the problem involving Intersection Chords Theorem?

Two chords AB and CD of a circle intersects perpendicularly at the point P. The length of the segments AP, PB, CP and PD are given. Can you calculate the radius of the circle? Watch the full video for an interesting solution with the help of intersecting chord theorem? Learn something new and different.

Can you solve the system of non-linear equation?

A system of linear equations can be solved easily by many standard methods. Even in high school mathematics courses, students are taught Cramer's rule and the method of Matrix to solve a given set of consistent linear equations up to three variables. More advance methods are taught in the course of linear algebra. But for a given system of non-linear equations, there is no specific method. Different ideas need to be applied across various parts of mathematics to get the solution. Nonetheless using school level mathematics, many interesting problems can be solved. Here we present once such problem, where we have to solve a system of non-linear equation. x^2 -  yz = 3, y^2 - zx = 4 and z^2 - xy = 5 is the system here, we will solve it using elementary ideas. Knowledge of arithmetic progression is essential.

Reach me:

My Blog: http://mathsvinu.blogspot.com

Twitter: http://twitter.com/prime_maths

Facebook: https://www.facebook.com/PrimeMaths

Instagram: https://instagram.com/prime.maths

Whatsapp: +91-9038126497 ( for Homework and assignment help )

Can You Solve The 5 Circles inside the square Problem?

Five circles with the same radius are placed inside a square, whose sides are not known. The radius of the circle is given. The problem is to find the side of the square. Four circles are placed inside the square at the four corners in such a way that the sides of the square are tangents to the circle. The fifth circle is place between the four circles, such that it touches the four circles externally.

Watch the video for the solution

Solved Problems : Circles for Class X

Solved Problems: Trigonometry - Angles and Sides of a Triangle.

To get the pdf file visit the page: http://kolkatamaths.yolasite.com/xi-xii.php
File name: Angles and Sides of a Triangle_Loney

Trigonometry: Elimination Problem

Solving a system of in-equations: A problem form High School

In this video, I have solve a system of in-equations, using elementary ideas of high school mathematics. Using the fact that, square of any real number is always greater than equal to zero, we can at the same time frame and solve unique problems. Watch the full video, to learn.